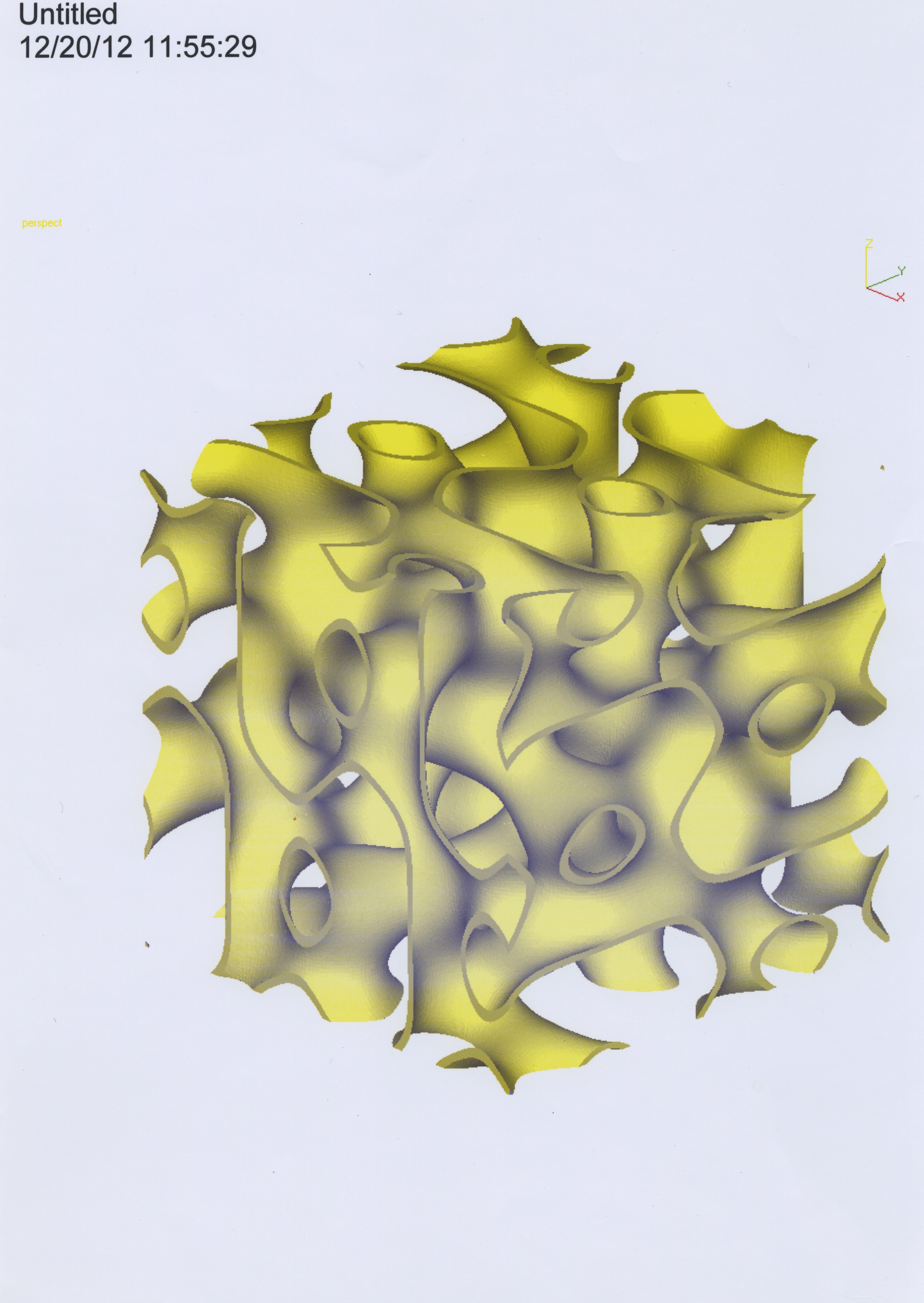
“Superficial minimalism” but look deeper!

These periodic minimal surfaces are two-dimensional universes or manifolds which are everywhere saddle-shaped. They have two principal curvatures which are everywhere equal and opposite. The examples shown have no arbitrary parameters but represent cubic building blocks which can be stacked together indefinitely to separate the world into two infinite sub-spaces, which in some cases may be the same and in some cases different. They can also be layered, giving many parallel non-intersecting universes.

These two-dimensional manifolds are non-Euclidean, in that the perimeter of a small circle of given radius on the surface is greater than that of one of the same radius on the plane (which in turn is greater than one of the same radius on the surface of a sphere). A gecko exploring the surface would find its world to be very complex in its labyrinthine interconnections. There are many ways of making a journey and then finding oneself back at the starting point. A two-dimensional gecko-world T. S. Eliot might well write:

“We shall not cease from exploration
And the end of all our exploring
Will be to arrive where we started
And know the place for the first time.”

Beyond the representation of the human figure, 3-D sculpture is often disappointing, but architecture is advancing, continually escaping from orthogonality towards the curved surfaces which modern technology makes practicable. Cézanne said: “Treat nature in terms of the cylinder, the sphere, the cone, all in perspective”, but we have moved on. Barbara Hepworth was renowned for being thought the first sculptor to make a hole through a piece of stone in an abstract design, but artists now have many more interesting topologies than that of the torus on which they can impose their superficial patterns.